How To Find The Moment Of Inertia Of An I Beam
Your guide to SkyCiv software - tutorials, how-to guides and technical manufactures
Moment of Inertia of a Circumvolve
Moment of Inertia of a Circle – A detailed breakup
Moment of Inertia is an important geometric property used in structural engineering, as it is directly related to the amount of fabric strength your section has. Generally speaking, the higher the moment of inertia, the more than strength information technology has and the less it will deflect under load. The Moment of Inertia of a circumvolve, or any shape for that matter, is essentially how much torque is required to rotate the mass almost an axis – hence the word inertiain its name. Note, this is not to be confused with Moment Area of Inertia (Second moment of inertia) which is a different calculation and value birthday.
The moment inertia is important for both Angle Moment Force/Stress and Deflection. This is evident considering their formula, wherein both cases I (Moment of Inertia) is in the denominator:
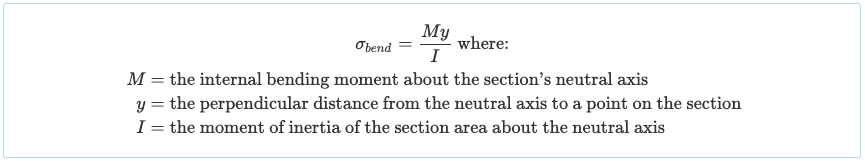
Source: Bending Stress Formula
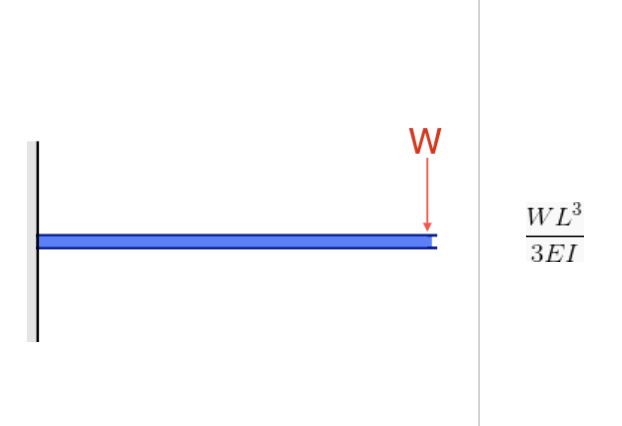
Source: Equation of Deflection in a Cantilever Beam
Moment of Inertia in round cross sections have a particular behaviour. Firstly, they accept the same moment of inertia in both axis (known as major and modest axis). This makes sense as the section is symmetrical in both the Ten and Y directions. We'll look at how this is not always the case in other sections, when nosotros compare with an I beam beneath. Withal, this can be a do good when loading is not always along the member's strong centrality, as yous can predict the force of the member regardless of the load management. Despite this, circular sections typically don't have very high moment of inertia values for their weight (in comparing to say an I beam for case) as we'll learn more in the next session.
Pros and Cons of Circular Sections
It's interesting to compare the moment of inertia of a circle compared to other shapes, to really empathise how it behaves differently. For one, most of the mass is concentrated effectually the centroid, with not as much mass at the meridian and lesser. This is pretty of import for moment of inertia calculations, since the further away the mass, the college the value. Allow'south look at a comparing, produced past SkyCiv'south Department Builder:
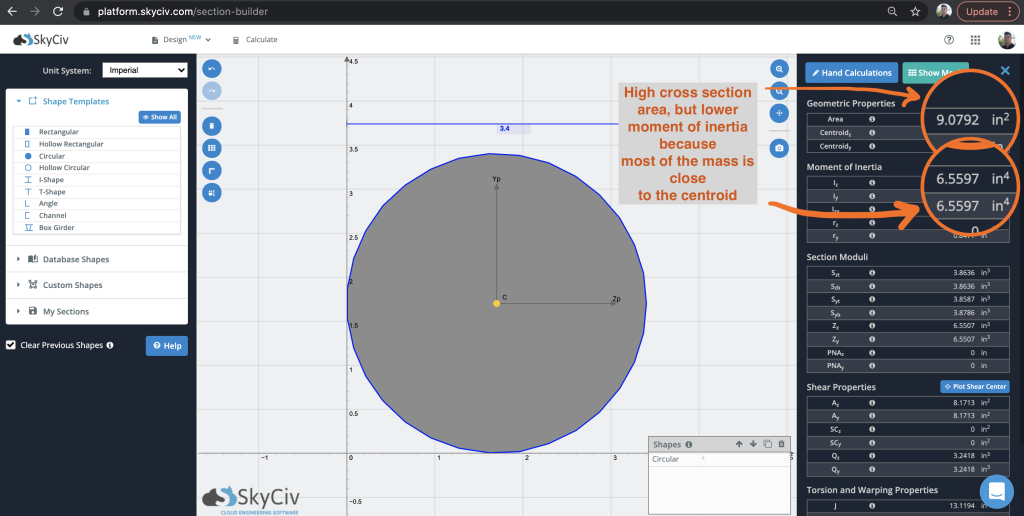
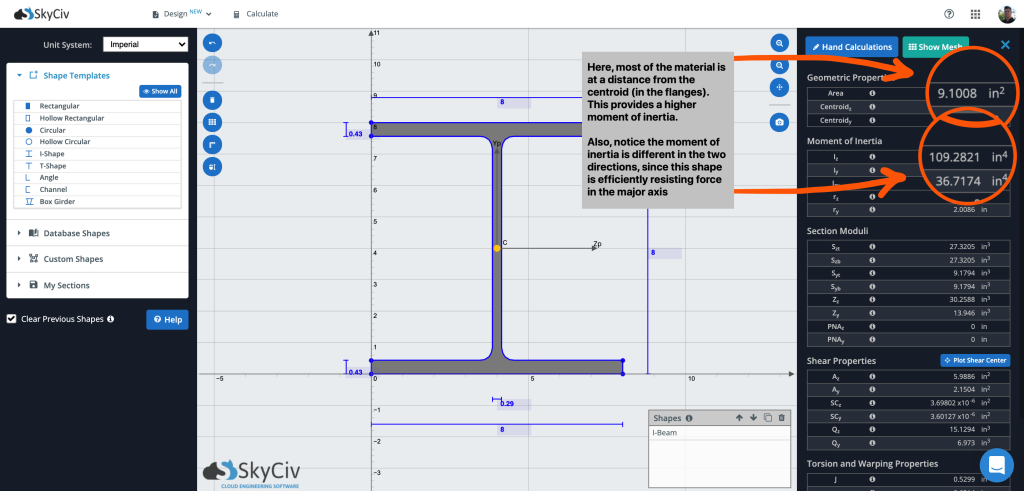
So in the above, nosotros have roughly 9 square inches of material providing a moment of inertia of 6.5597. We tin also see that the Iy and Iz values are the same because the department is symmetrical in both directions, equally previously mentioned. When comparing this to an I-Beam with the same area, nosotros can see the difference of having almost of our mass further abroad from the centroid:
Moment of Inertia of a Hollow Circular Section
Given this behaviour, this is frequently why we don't see many solid circular sections in structural applied science and are often replaced with more favourableHollow Round sections. These are more efficient at providing higher moment of inertia values for the same reason every bit the I beam: most of the mass is at a altitude from the centroid. Consider a hollow round shape with like area:
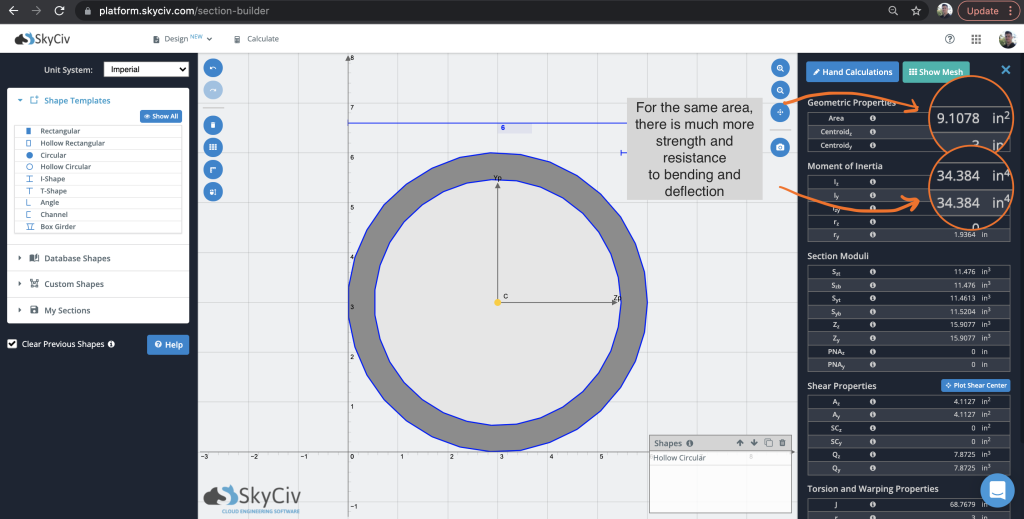
And so, in summary for the same textile equally a solid circular section, the moment of inertia is over 5 times stronger. This plays a critical role in the strength against bending moment strength and deflection.
Moment of Inertia of a Circle Formula
Another useful exercise is to wait at this is all by considering the full general moment of inertia circumvolve formula:
[math] I_{ten}, I_{y}=\dfrac{\pi}{64}D^4 [math]
And the moment of inertia formula for hollow circular sections:
[math] I_{x}, I_{y}=\dfrac{\pi}{64}D^4 – \dfrac{\pi}{64}d^four [math]
Obviously, nosotros can run into that some of the moment of inertia is removed from the cutout. However, because this is nor providing much restraint against angle (given it'due south and then shut to the centroid), information technology is an inefficient utilize of material. So removing this part of the section really improves the efficiency of the department.
Try SkyCiv Moment of Inertia Calculator For Free
Most of this article was written with the assistance of SkyCiv's total Section Builder Software, we also offer a free version and so y'all tin can experiment with these calculations:
Source: https://skyciv.com/docs/tutorials/section-tutorials/moment-of-inertia-of-a-circle/
Posted by: freythum1941.blogspot.com

0 Response to "How To Find The Moment Of Inertia Of An I Beam"
Post a Comment